Introduction to volume formula
Understanding volume formula is crucial for many aspects of our lives, from baking a cake to designing a room. Whether you’re an enthusiastic student or just someone curious about the world around you, grasping how to calculate the space within 3D shapes can be both valuable and fun. Volume formulas help us make sense of three-dimensional objects by determining how much space they occupy.
But don’t worry if math isn’t your strong suit! This guide will briefly break down the key concepts related to volume formula . We’ll explore common 3D shapes and their formulas while providing practical examples demonstrating why understanding volume matters in everyday situations. Get ready to dive into the fascinating world of geometry and unlock the secrets behind those seemingly complex calculations!
Common 3D Shapes and their Formulas
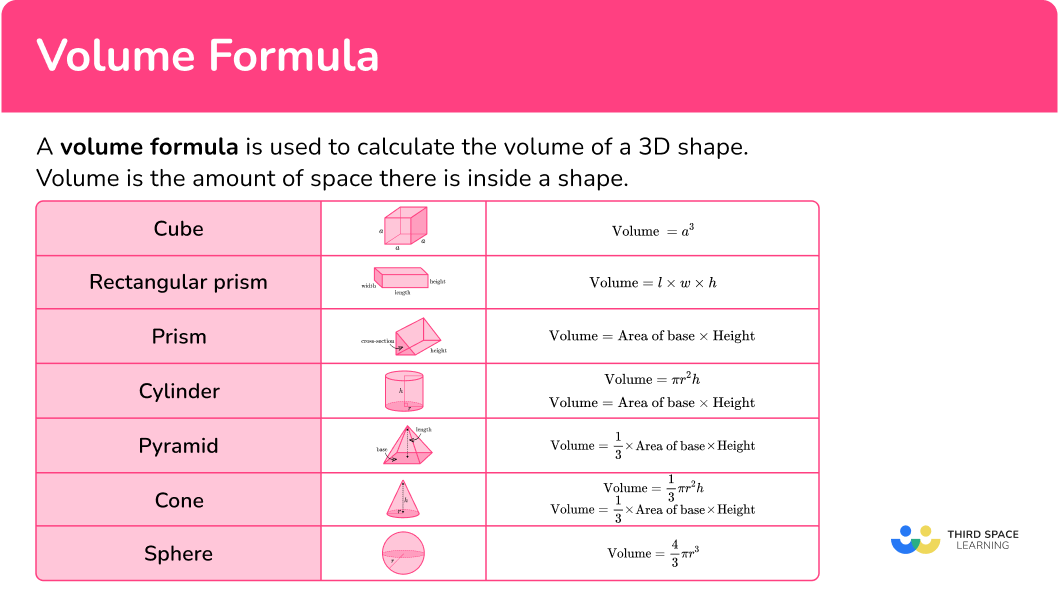
When diving into the world of volume formulas, it’s essential to understand common 3D shapes. Each shape has its unique formula that makes calculating volume straightforward.
Let’s start with cubes and rectangular prisms. The formula is simple: length × width × height. This applies whether you’re working with a box or a square block.
Next up are cylinders, often seen in cans and tubes. Their volume formula can be found using the formula πr²h, where r is the radius at the base and h is the height.
Cones have a slightly different approach. To find their volume formula , use (1/3)πr²h. This gives you an idea of how much space fits inside those ice cream cones we all love.
Spheres are captivating in their symmetry. The volume formula for spheres utilizes the formula (4/3)πr³ to determine how much room exists within these perfectly round shapes.
– Cubes and Rectangular Prisms
Cubes and rectangular prisms are foundational shapes in geometry. Both have straight edges and flat surfaces, making them easy to visualize.
A cube is a particular type of rectangular prism. All six faces of a cube are squares with equal side lengths. This symmetry makes calculations straightforward.
The volume formula for a cube is simple: V = s³, where “s” represents the length of one edge. Just multiply the side length by itself three times.
Rectangular prisms, on the other hand, can come in various dimensions. Their volume formula formula is slightly different: V = l × w × h, where “l” stands for length, “w” for width, and “h” for height.
Both shapes, from packaging boxes to buildings, can be found everywhere, showing their practical importance in daily life. Understanding these formulas helps us appreciate the structure around us more deeply.
– Cylinders
Cylinders are fascinating 3D shapes consisting of two parallel circular bases connected by a curved surface. Think about those classic soda cans or sturdy pipes we see every day. Their uniform shape makes them both practical and easy to visualize.
To find the volume formula of a cylinder, we use a simple formula: V = πr²h. Here, “V” represents the volume, “r” is the radius of one base, and “h” is the height of the cylinder. This straightforward equation allows us to calculate quickly how much space lies within these structures.
Imagine filling a cylindrical container with water or sand; understanding this formula helps you determine its capacity. Cylinders are widely used in various industries—from construction to packaging—so grasping their volume formula calculations becomes essential for many applications.
– Cones
Cones are fascinating 3D shapes that taper smoothly from a flat base to a pointed tip, known as the apex. You can find cones in everyday objects, like ice cream cones or traffic cones.
To calculate the volume formula of a cone, you use the formula V = (1/3)πr²h. Here, “r” represents the radius of the base while “h” signifies its height. This formula captures how much space is inside your cone.
Understanding this shape’s properties helps demystify its role in geometry and real-life applications. For instance, when pouring liquids into a conical cup, knowing its volume formula ensures you don’t spill over.
Visualizing this shape can be easier with hands-on activities—like making paper cones! Crafting them yourself will help youwillyou’llyou intuitively grasp their dimensions and volume formula .
– Spheres
Spheres are perfectly symmetrical 3D shapes. They are definedThey’They’rened as the set of all points in space equidistant from a central point. This unique characteristic gives spheres their smooth, round appearance.
The volume formula formula for a sphere is derived from its radius. The equation is V = (4/3)πr³, where r represents the radius. It’s It’sinatingtionship captures the essence of such a complex shape.
Imagine holding a basketball or gazing at Earth from space; both are examples of spheres in action. Their uniformity allows them to roll smoothly and distribute weight evenly.
Understanding the geometry behind spheres opens up new perspectives in physics and engineering. From designing sports equipment to studying planetary motion, the significance of volume formula formulas must be considered when designing spherical objects.
Calculating the volume formula Step-by-Step
Calculating volume formula can initially seem daunting, but breaking it down into steps makes it manageable. Start by identifying the shape you are with.
For a cube, tyou’ryou’reinghe formula is simple: V = s³. Just measure one side and raise it to the power of three.
Rectangular prisms use V = l × w × h. Measure length, width, and height; multiply those values for your answer.
Cylinders require a bit more work: V = πr²h. Determine the radius of the base and height before plugging them into this formula.
It’s similar forties:It’s (1/3)πr²h. The extra factor of one-third sets this apart from cylinders.
Spheres are calculated using V = (4/3)πr³. Measuring the radius allows you to find its volume through exponentiation and multiplication with pi.
Take your time with each step for accuracy!
Real-World Applications of Volume Formulas
Volume formulas have practical significance across various fields. Architects rely on these calculations when designing buildings, as they need to understand how much space their structures will occupy.
In the culinary world, chefs use volume measures to create recipes. Accurate portions ensure that flavors blend perfectly in every dish.
Manufacturers also depend on volume computations to efficiently package products. Knowing the right dimensions helps minimize waste and reduce costs.
Environmental scientists utilize volume formulas to assess natural bodies of water. This information is crucial for managing ecosystems and understanding aquatic habitats.
Students frequently encounter these concepts in physics experiments. Understanding volume aids in effectively grasping principles like buoyancy or displacement.
From everyday tasks to complex scientific studies, mastering volume formulas unlocks a wealth of knowledge that is applicable everywhere!
Tips for Remembering Volume Formulas
Remembering volume formulas can be challenging, but a few tricks can make it easier.
Use mnemonic devices to create memorable phrases or acronyms. For example, for the formula of a sphere (V = 4/3πr³), think of “Four “outta three pi”zas r”und.” This” silly phrase “inks “umbers and shapes in your mind.
Visual aids can also help. Draw each shape with its corresponding formula nearby. Associating visual elements with text reinforces memory retention.
Practice is another effective method. Regularly solving problems using these formulas will solidify them in your brain over time.
Engage with interactive tools online that allow you to manipulate shapes visually while calculating volumes simultaneously.
Teach someone else what you’ve done! Explainiyou’vecepts helps reinforce your understanding and keeps the information fresh in your memory.
Alternative Methods for Finding Volume
Finding volume doesn’doesn’ts have tdoesn’doesn’tsulas. There are alternative methods that can simplify the process.
Water displacement is a practical approach for irregular shapes. By submerging an object in water and measuring the rise in liquid level, you can determine its volume accurately.
Another method involves modeling clay or other malleable materials. Mold your shape and then compare it to known volumes of similar geometric solids to estimate the volume.
If you’re tech-savvy, coyou’re 3D scanning software. This technology captures detailed measurements of objects and computes their volume within seconds.
Approximation techniques come into play when precision isn’t necessary. Estiisn’tg based on nearby shapes allows quick calculations without diving into complex math equations. Experiment with these options to discover what works best for you!
Conclusion
Understanding volume formulas is essential for anyone dealing with 3D shapes in academic settings or everyday scenarios. We can demystify how these shapes occupy space by breaking down the formulas related to cubes, cylinders, cones, and spheres.
Calculating volume can be manageable. With clear steps and understanding each formula’s competencieformula’s can master this skill. Real-world applications range from architecture to cooking; knowing how much space an object occupies can make a significant difference.
Remembering these formulas may seem tricky at first. However, employing mnemonic devices or visual aids can help solidify your knowledge. Additionally, exploring alternative methods such as displacement or using technology like 3D modeling software provides multiple avenues for finding volume.
Mastering the concept of volume opens doors to practical problem-solving in various fields. Whether you’re a student aimiyou’re better grades or a professional needing accurate calculations in your work life, grasping these concepts will significantly enhance your confidence and capabilities when working with 3D shapes. Embrace the challenge and let it enrich your understanding of our three-dimensional world.




